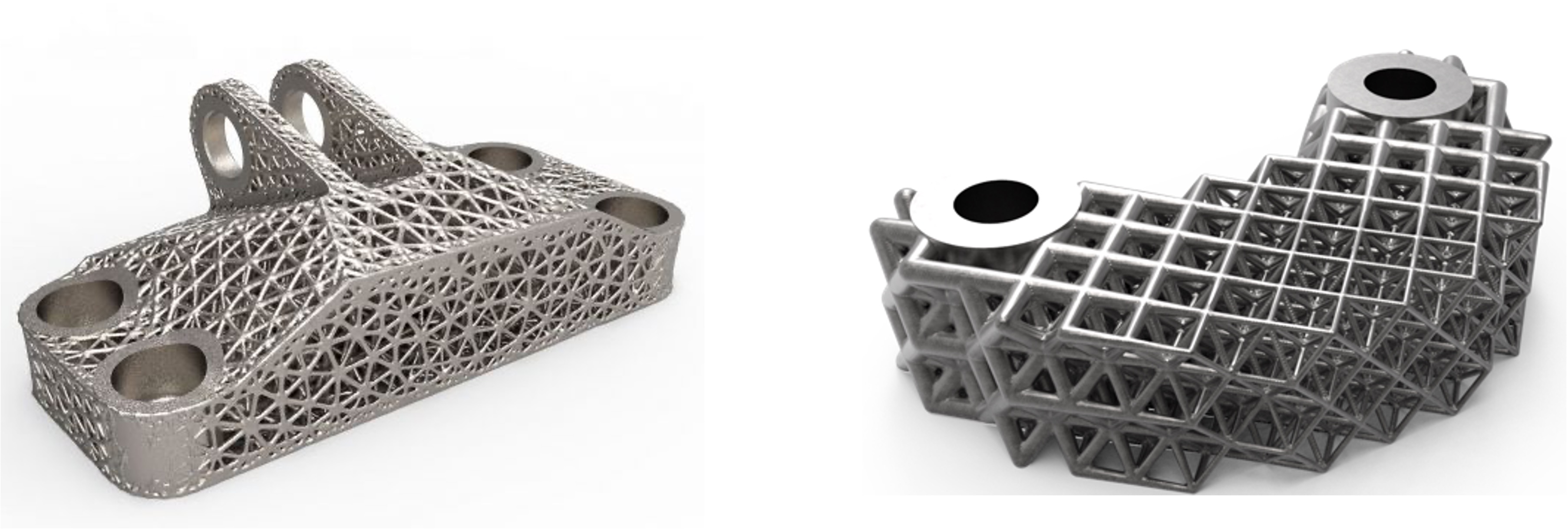
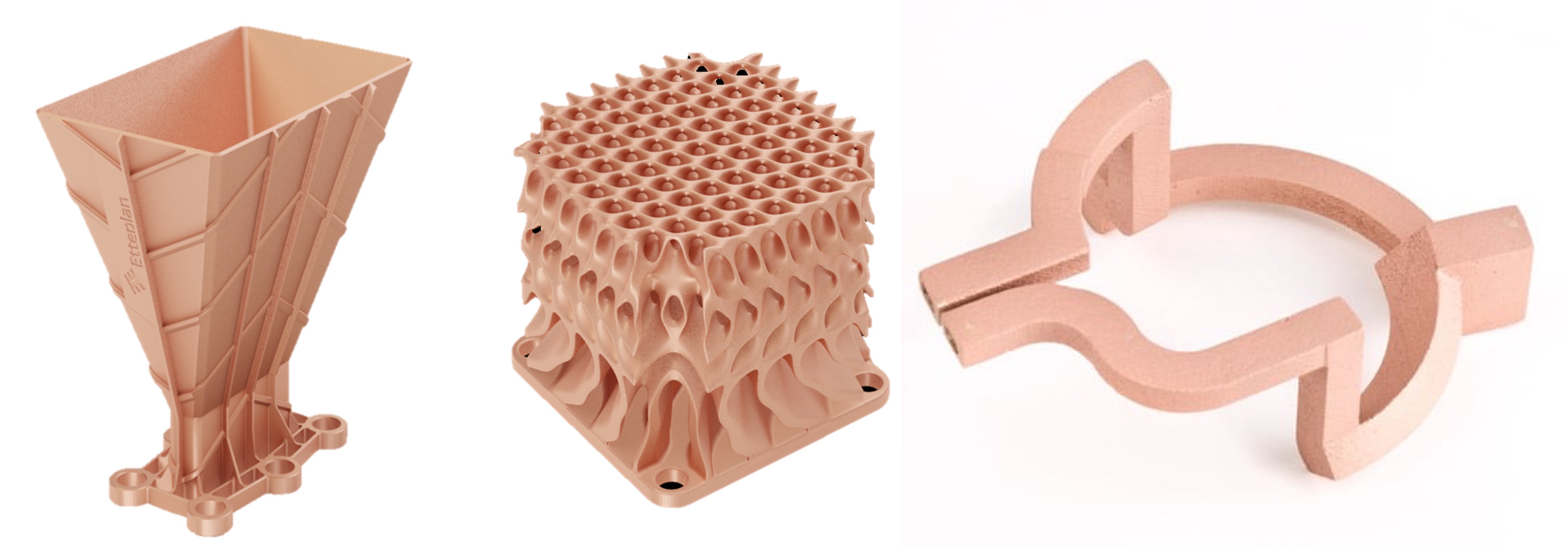
Cellular lattice structures are becoming a more common feature of parts designed for additive manufacturing. Cellular lattice structures can exhibit a combination of high strength, relatively low mass, good energy absorption characteristics and good thermal and acoustic insulation properties. However, lattice structures are difficult to manufacture via conventional methods such as CNC machining, injection molding, investment casting, brazing or metal wire approaches. Conventionally manufactured lattices are limited to simple geometries, with relatively large dimensions compared to the critical dimensions required for use in many advanced metal components.
Body centred cubic(BCC) Face centred cubic(FCC) Hexagonal centred cubic(HCC)
Lattice structures in AM
Honeycomb structure
A hexagonal honeycomb is a pattern with hexagonally shaped walls, all orthogonal to the same plane. This is a common lattice structure for the infill of FFF/FDM parts, and for some SLA parts such as sacrificial patterns used in investment casting. The in-plane stiffness and strength of honeycombs are lowest because stresses in this plane make the cell walls bend. The out-of-plane stiffnesses and strengths are much larger because they require axial extension or compression of the cell wall.
When a honeycomb structure is being deformed in a plane, it broadly exhibits a linear-elastic regime followed by a plateau of roughly constant stress, leading into a final regime of steeply rising stress. The linear-elastic regime is due to bending of the cell walls, but when critical stress is reached the cells begin to collapse resulting in the plateau region and eventually at high strains, the cells collapse sufficiently that opposing cell walls touch resulting in densification and steep increase in the stress. A widespread application of honeycomb lattices made using traditional manufacturing techniques is the reinforcement of load-bearing structural panels, and the flexibility of AM to tailor the dimensions of the honeycomb cells according to local loading conditions offers new opportunities in these applications.
Bending of cell walls due to linear-elastic property
3D Printed honeycomb structure & honeycomb sandwich structure
Open-and Closed-Cell foams
Three-dimensional foam structures can be open-cell or closed-cell structures and be regular or random in their arrangement. Synthetic foams, manufactured on a large scale, are used for absorbing mechanical impact energy, and managing weight-efficient strength, thermal conductivity, and buoyancy. The important features of foam are relative density, the degree to which the cells are open or closed, and the extent of anisotropy.
The cell shapes in real foams are more complex than regular open-cell structures. However, the modes of deformation are similar such that the scaling of mechanical properties can be understood by using dimensional arguments that are independent of the geometry. Open-cell lattices can have many topologies, defined by the arrangement of the beams in the unit cell, and how the unit cells are arranged and attached to one another. Two important categories of open-cell lattices are those with mechanical behaviour dominated by bending, and by stretching.
Cell deformation under force. Source: Gibson and Ashby
Bend-dominated lattice Stretch-dominated lattice
Stretch-dominated lattices are much stiffer and stronger than bend-dominated lattices at the same relative density because the horizontal beam across the midline of each unit cell reinforces the cell as it is compressed and is thereby loaded in tension.
Auxetic Lattices
Auxetic lattices are special periodic geometries that exhibit unconventional, nonlinear mechanical behaviour. The most common auxetic structure is the inverted honeycomb, which has a negative Poisson’s ratio. In other words, when it is stretched along one axis, it expands along the other axis; conversely, when compressed along one axis, it densifies along the other axis. Other structures include dilational metamaterials, inverted Kelvin Cells, Buckley crystal, buckling induced patterns. Like periodic open and closed lattice structures, the mechanical behaviour of auxetic lattice structures is heavily dependent on the dimensional parameters yet is more difficult to predict using conventional scaling methods.
One application of auxetic structures made by AM is to tailor the thermal expansion properties and energy absorption of structures by design. For instance, auxetic lattices made of multiple materials can be designed to exhibit zero or negative effective thermal expansion. The local densification upon compression also favours localization of impact energy, e.g., for lightweight armour materials. These nonlinear properties are also of interest for printed fabrics, medical braces, and shoes.
Credit: nTopology
Video credits: MIT
Applications of lattice structures
Lattice structured sole of a shoe. Photo credits: Carbon3D
Architecture design. Photo credits: Lilian van Daal
Multi-purpose applications
Conclusion
- Lattice structure can enhance or improve the performance of an existing system or a new product
- Lattice geometry is the key tool for the creation of lightweight structures
- Lattice structures made my AM enable the creation of complex and optimized designs in different materials
- These complex lattice structures cannot be created by the conventional manufacturing process
- Various real-world examples have validated that major improvements in strength and stiffness could be realized thru uneven unit cells and functionally graded designs.
Future work
- Metal AM processes need further improvements to accurately print lattice-type structures
- A better understanding of fatigue properties and scaling effects of the lattices is needed. These properties are more sensitive to surface defects.
- Optimization of lattice structures thru numerical designs and best practices are not fully established.
Blog created by - 3D SculpLab Team


 3D SculpLab
3D SculpLab